Invertible System
The system is invertible if by observing the output, we can determine its input i.e. we can construct an inverse system that when cascaded with the given system, as shown in figure1, yields an output equal to the original input of the given system.
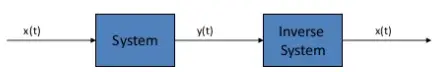
The inverse system ‘undoes’ what the given system does to input.
When several different inputs result in the same output, it is impossible to obtain the input from the output and system is non-invertible.
For an invertible system, it is essential that every input has a unique output so that there is one to one mapping between an Input and the corresponding output.
A rectifier, specified if by an equation y(t) = |x(t)|, is non-invertible because the rectification operation cannot be undone.
